Triangle geometry draw centroid coordinate median
Table of Contents
Table of Contents
The centroid is an important concept in geometry that is used to determine the geometric center of a shape. Drawing a centroid can be a challenging task for many individuals but is crucial for students and professionals in various fields who need to calculate the centroid of compound shapes or design composite materials that can resist different loads.
Understanding how to draw a centroid is essential for engineers, architects, and designers who need to calculate moments of inertia and other physical properties of irregular shapes. However, many people find this task daunting, as it requires significant knowledge of basic geometry, trigonometry, and calculus.
The process of drawing a centroid involves several steps, but it can be simplified into a specific formula. The formula involves calculating the average of the x-coordinates and y-coordinates of a shape’s vertices. The resulting point is the centroid of the shape.
In summary, understanding how to draw a centroid is essential for anyone involved in engineering, architecture, or design. Drawing a centroid is a process that can be simplified into a specific formula. The formula involves calculating the average of the x-coordinates and y-coordinates of a shape’s vertices.
How to Draw a Centroid: Understanding the Formula
When I was in engineering school, I struggled with grasping how to calculate the centroid of complex shapes. I found myself trying to apply the formula to various shapes, but I kept getting the wrong answers. One day, my professor showed us a simple trick that helped me grasp the concept of drawing a centroid.
The trick involved splitting the shape into smaller shapes and then calculating the centroid of each small shape. By summing all the centroid points and dividing by the total area, we could determine the final centroid of the shape.
The formula to draw a centroid involves calculating the average of the x-coordinates and y-coordinates of a shape’s vertices. In other words:
Centroid = [(x1 + x2 + x3 + … + xn) / n , (y1 + y2 + y3 + … + yn) / n]
where:
- (x1, y1), (x2, y2), (x3, y3),…, and (xn, yn) are the coordinates of the vertices of the shape
- n is the total number of vertices in the shape.
The formula is straightforward, but it requires some knowledge of algebra and calculus to apply correctly. However, with some practice and patience, anyone can learn how to draw a centroid.
Using the Centroid to Determine Moments of Inertia
One critical application of centroids is in determining the moment of inertia of a shape. Understanding how to calculate the moment of inertia is essential for designing structures and machines to withstand different loads and forces.
The moment of inertia of a shape is the resistance to rotating the shape about an axis. The formula for calculating the moment of inertia of a shape about an axis is:
I = ∫(y^2)dA
where:
- I is the moment of inertia about the axis
- y is the distance from the axis to an infinitesimal element of area dA.
The moment of inertia can be calculated using integration techniques, but it is often easier to use the parallel axis theorem. The theorem states that:
I = Icm + AD^2
where:
- Icm is the moment of inertia about the centroidal axis
- A is the area of the shape
- D is the distance between the centroidal axis and the new axis of rotation.
This formula makes it easier to calculate the moment of inertia about a new axis of rotation without having to integrate the entire shape. By knowing how to draw a centroid and using the parallel axis theorem, designers can quickly determine the moment of inertia of a shape, which is an important factor in designing structures, machines, and other systems.
The Importance of Learning How to Draw a Centroid
As we’ve seen, drawing a centroid is an essential skill for anyone studying or working in engineering, architecture, or design. Understanding how to draw a centroid is the foundation for many other concepts, such as moment of inertia, center of gravity, and others.
By learning how to draw a centroid and other related concepts, individuals can increase their technical skills and knowledge, making them more proficient in their work. Additionally, mastering these concepts can lead to better job opportunities and salary prospects in various industries.
Questions and Answers
Q: What is a centroid?
A: A centroid is the geometric center of a shape. It is the point where all the lines of symmetry of the shape intersect.
Q: Why is the centroid important?
A: The centroid is important because it is used to determine various physical properties of objects, such as moment of inertia, center of gravity, and others.
Q: How do you calculate the centroid of an irregular shape?
A: To calculate the centroid of an irregular shape, you need to divide the shape into smaller shapes and then calculate the centroid of each small shape. Afterward, you need to sum all the centroids and divide by the shape’s total area.
Q: How is the centroid used in engineering?
A: The centroid is used in engineering to determine various physical properties of objects, such as moment of inertia, center of gravity, and others. By knowing these properties, engineers can design structures and machines that can withstand different loads and forces.
Conclusion of How to Draw a Centroid
Drawing a centroid can be a challenging task, but it is an essential skill for anyone studying or working in engineering, architecture, or design. By understanding the formula for calculating the centroid of a shape and how to use it to determine the moment of inertia, individuals can increase their technical skills and knowledge, making them more proficient in their work. Ultimately, knowing how to draw a centroid leads to better job opportunities and salary prospects in various industries.
Gallery
Centroid Of Various Section - YouTube
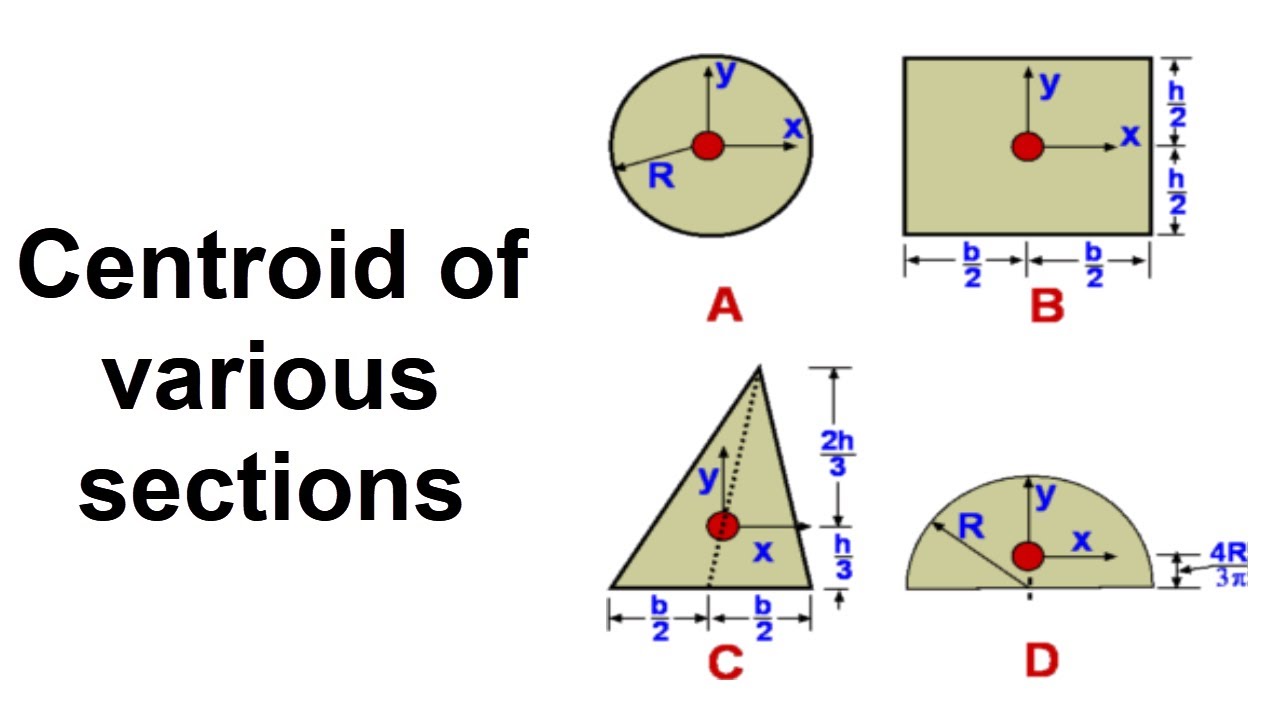
Photo Credit by: bing.com / section
Triangles - Technical Graphics
Photo Credit by: bing.com / centroid triangle find triangles technical graphics weebly
Draw An Isosceles Triangle. Locate Its Centroid, Orthocentre
Photo Credit by: bing.com / centroid isosceles orthocentre collinear verify sarthaks its lie
What Is Mean By Centroid Of A Triangle…how To Find Centeroid Of A
Photo Credit by: bing.com / triangle geometry draw centroid coordinate median
Calculating The Centroid Of Compound Shapes Using The Method Of
Photo Credit by: bing.com / centroid calculator calculating centroids owlcation centroide deposition decomposition
